Lot Size Optimization

Efficient order quantities (EOQs) incorporate the cost to place an order (POC), the forecasted annual demand (FAD), the inventory-carrying rate (ICR), and the unit inventory value (UIV). Large order quantities yield high inventory levels and high inventory carrying costs but fewer orders and lower ordering costs. High ordering costs and demand rates suggest large order quantities. High inventory carrying rates and high unit inventory values suggest small order quantities.

The economic order quantity (EOQ) is the lot size that minimizes the total cost of ordering and carrying the inventory associated with the order. The higher the order quantity, the greater the inventory level. However, the higher the order quantity the fewer the number of orders and the lower the resulting ordering cost. The economic run quantity (ERQ) is the production lot size (or run quantity) that minimizes the total of setup/changeover costs and the inventory carrying costs associated with the inventory produced by the run length. The tradeoffs between manufacturing setup cost and inventory carrying costs for determining optimal production run sizes for a large textiles client are illustrated. Note in the example that the optimal run length is 3 or 4 rolls per setup for that particular SKU. As is often the case with EOQ modeling, the total cost curve is fairly flat near the optimal solution. The key, as is often the key, is to make decisions that are at least in the “ballpark of optimal”. Unfortunately we often find that lot sizing is off by 200% or 300%.

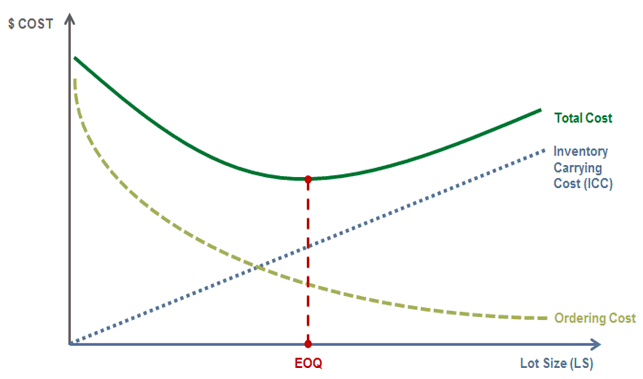
Lot Size Optimization Curve

The formula to compute the EOQ for a purchased item is as follows:

EOQ = {(2 x FAD x POC) / (UIV x ICR)}^1/2

For example, if an item has an annual demand of 3,000 units per year; a purchase order costThe purchase order cost (POC) is the cost of placing a purchase order from a vendor. Those costs include: Order Forms Postage Telecommunications Authorization Purchase Order Planning Purchase Order Entry Time Purchase Order Processing Time Purchase Order Inspection Time Purchase Order Follow-up Time Purchasing Management Office Space Office Supplies Purchase Order Entry Systems Tracking and Expediting The most expensive items on the list are the labor related items. Hence, automating purchase order processing typically yields significant labor cost reductions and productivity improvements. of $300 per purchase order; a purchase price of $2,100 per unit; and an inventory carrying rate of 30% per year then its EOQ is

EOQ = [(2 x 3,000 x $300)/($2,100 x 30%)] ^½ = [(1,800,000)/(630)] ^½ = [2,857]^1/2 = 53 units

The formula to compute the EOQ for a manufactured item, sometimes referred to as the economic run quantity (ERQ) is as follows:

EOQ = {(2 x FAD x SUC) / (UIV x ICR)}^1/2

For example, if an item has an annual demand of 5,000 units per year; a setup cost of $3,200 per setup; a standard cost of $85.00 per unit; and an inventory carrying rate of 25% per year then its EOQ is

EOQ = [(2 x 5,000 x $3,200)/($85 x 25%)] ^½ = [(32,00,000)/(21.25)]^½ = [1,505,882]^1/2 = 1,227 units

EOQ is considered passé, outdated, and nearly pre-historic in many inventory circles. Yet, in our work with the most advanced supply chain organizations around the world we are finding great profit, service, and operational improvements with EOQ.

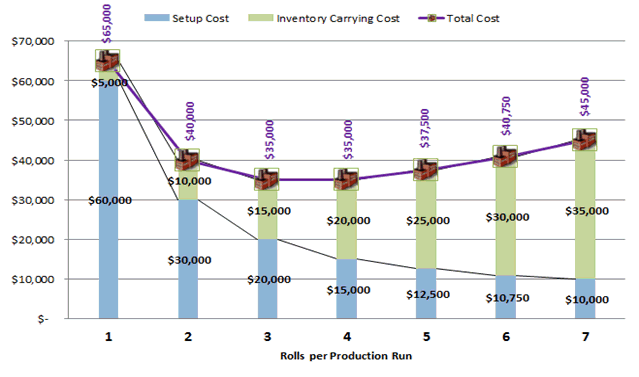
RightChain™ Lots Optimization for a Major Textiles Company